Difference between revisions of "804: Pumpkin Carving"
m (removed Category:Axiom of Choice using HotCat) |
(→Explanation) |
||
| Line 20: | Line 20: | ||
Black Hat, rather unconvincingly, insists that his pumpkin is suffering from chest pains, and that he is merely treating these pains with nitroglycerin. While it is true that this chemical is used to treat {{w|angina}} (chest pain due to blocked arteries in the heart), nitroglycerin used for this purpose is dispensed in small spray bottles and controlled by prescription. Also, pumpkins are a vegetable and do thus not contain nervous or circulatory systems of mammalian complexity; even if they did, the process of pumpkin carving involves hollowing them out, making it a moot point. | Black Hat, rather unconvincingly, insists that his pumpkin is suffering from chest pains, and that he is merely treating these pains with nitroglycerin. While it is true that this chemical is used to treat {{w|angina}} (chest pain due to blocked arteries in the heart), nitroglycerin used for this purpose is dispensed in small spray bottles and controlled by prescription. Also, pumpkins are a vegetable and do thus not contain nervous or circulatory systems of mammalian complexity; even if they did, the process of pumpkin carving involves hollowing them out, making it a moot point. | ||
| − | To top it off, Black Hat plans to put up a sign warning passers-by to not smash the pumpkin. This would only serve to tempt impulsive teenagers to disturb it, which is very likely what the sadistic and chaos-loving [[72: Classhole|Classhole]] is hoping for. If he succeeds with his plan, with a completely hollowed out pumpkin of the shown size filled with nitroglycerin it would seem likely that he would kill a teenager that | + | To top it off, Black Hat plans to put up a sign warning passers-by to not smash the pumpkin. This would only serve to tempt impulsive teenagers to disturb it, which is very likely what the sadistic and chaos-loving [[72: Classhole|Classhole]] is hoping for. If he succeeds with his plan, with a completely hollowed out pumpkin of the shown size filled with nitroglycerin it would seem likely that he would kill a teenager that stomped on it, or at least would blow off a leg. In either case a punishment that most people would not consider befitting of such a petty crime. |
[[Randall]] was out in time with this comic for someone to try this out at home when Halloween fell 20 days later. It has been known (particularly by Randall) that people copy his ideas... See for instance this earlier [http://xkcd.com/chesscoaster/ post] on xkcd based on [[249: Chess Photo]]. Soon after he even made a comic, [[254: Comic Fragment]], that was supposed to be impossible to copy, which he mentioned him self later (see the explanation). So he must have been expecting someone to try this one out... | [[Randall]] was out in time with this comic for someone to try this out at home when Halloween fell 20 days later. It has been known (particularly by Randall) that people copy his ideas... See for instance this earlier [http://xkcd.com/chesscoaster/ post] on xkcd based on [[249: Chess Photo]]. Soon after he even made a comic, [[254: Comic Fragment]], that was supposed to be impossible to copy, which he mentioned him self later (see the explanation). So he must have been expecting someone to try this one out... | ||
Revision as of 18:38, 3 October 2016
| Pumpkin Carving |
 Title text: The Banach-Tarski theorem was actually first developed by King Solomon, but his gruesome attempts to apply it set back set theory for centuries. |
Explanation
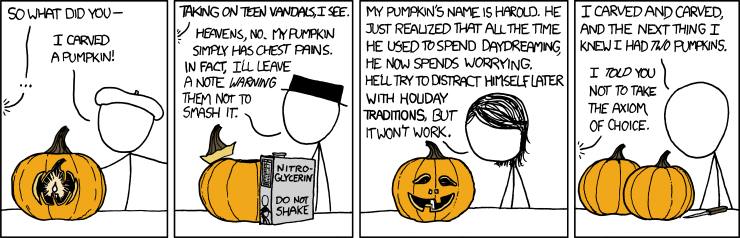
This comic is a reference to the custom of carving pumpkins to set out on porches and front steps for the holiday of Halloween, which occurs on October 31. (This comic was released 20 days before Halloween in 2010, maybe trying to give people some great ideas for their pumpkins.)
The pumpkin has the inside emptied out and a face or design carved in the side. Then a light is placed inside (usually a candle). These are called "Jack-O'-Lanterns". The Jack-O'-Lantern in the 3rd frame is the typical and standard design for a carved pumpkin.
The comic is set up as a typical TV program where an off-screen interviewer asks four (very) different people what they have made out of their Halloween pumpkin. In the official transcript the interviewer that talks in three of the panels is called an Interlocutor; "A person who takes part in dialogue or conversation."
In the 1st frame, Beret Guy, naturally, stays oddly on-topic by physically carving an image of a pumpkin in his pumpkin.
In the 2nd frame, Black Hat is shown with a box of nitroglycerin (a highly explosive liquid that may explode violently by just small bump) next to his pumpkin. He has not carved a hole for his lamp, but it seems he has emptied the inside of the pumpkin as the stem at the top has been removed. This will make it possible to fill up the pumpkin with nitroglycerin which would cause it to explode if agitated. Teenagers are a rather impulsive and rebellious lot[citation needed]; as Halloween is a night with lots of meticulously erected decorations and more lax parental supervision, troublemaker teens see it as an enticing time to engage in rampant vandalism, including but not limited to pumpkin-smashing. Hence, the off-panel character presumes that Black Hat is setting up a trap to get back at these ne'er-do-wells.
Black Hat, rather unconvincingly, insists that his pumpkin is suffering from chest pains, and that he is merely treating these pains with nitroglycerin. While it is true that this chemical is used to treat angina (chest pain due to blocked arteries in the heart), nitroglycerin used for this purpose is dispensed in small spray bottles and controlled by prescription. Also, pumpkins are a vegetable and do thus not contain nervous or circulatory systems of mammalian complexity; even if they did, the process of pumpkin carving involves hollowing them out, making it a moot point.
To top it off, Black Hat plans to put up a sign warning passers-by to not smash the pumpkin. This would only serve to tempt impulsive teenagers to disturb it, which is very likely what the sadistic and chaos-loving Classhole is hoping for. If he succeeds with his plan, with a completely hollowed out pumpkin of the shown size filled with nitroglycerin it would seem likely that he would kill a teenager that stomped on it, or at least would blow off a leg. In either case a punishment that most people would not consider befitting of such a petty crime.
Randall was out in time with this comic for someone to try this out at home when Halloween fell 20 days later. It has been known (particularly by Randall) that people copy his ideas... See for instance this earlier post on xkcd based on 249: Chess Photo. Soon after he even made a comic, 254: Comic Fragment, that was supposed to be impossible to copy, which he mentioned him self later (see the explanation). So he must have been expecting someone to try this one out...
In the 3rd frame, Megan is our typical emotional xkcd comic character. She is projecting herself onto the typical jack-o'-lantern she has carved (as the only one of the four), which she has even named Harold. It (or she) has been using a lot of time daydreaming and she has just realized that she now uses that time for worrying. She(it) now tries to distract herself with holiday traditions, but she already knows that it won't work. An example of a typical holiday depression.
In the 4th and frame, Cueball is shown in front of two un-carved pumpkins exclaming that he carved and carved in one and sudenly had two. He is here referencing the Banach-Tarski paradox (which is made clear in the title text), a theorem which states that it is possible to carve a three-dimensional ball, in this case a pumpkin, into a finite number of "pieces," and then reassemble these "pieces" into two different balls identical to the original. This paradox has been proven for just about anything theoretically, but requires infinitely complicated pieces, which are impossible for anything made of physical atoms rather than mathematical points.
The off-screen interviewer in that frame references the Axiom of Choice stating that Cueball should not have used that. The axiom says that given a set of buckets or bins, each containing one or more objects, it is possible to select exactly one object from each bucket. The Banach-Tarski rests on several axioms which are fairly well respected, but also requires the Axiom of Choice to work correctly. So a person who does not believe in the Axiom of Choice would not have been able to do what Cueball managed to do.
The title text says that King Solomon developed the Banach-Tarski theorem first. This is a reference to the story of two women being brought before him. Both were arguing that a particular child was their own. Solomon said that the solution was to cut the baby in half and give each woman one of the halves. One of the two women said that the other should have the baby whole. Solomon then knew she was the true mother, and gave her the child. The joke is that Solomon may have actually intended to cut the child, but, believing that two whole children could be made from the one, intended to give a baby to each woman, and the Banach-Tarski paradox states that, were the baby infinitely divisible, it should have been possible. As he of course failed completely in this process (so in Randall's version he actually did try to cut the baby), he set back the set theory for centuries, because he had "proved" the theorem wrong. The axiom of choice and set theory was later referenced in 982: Set Theory and, much later, the axiom of choice was mentioned again in the title text of 1724: Proofs.
Transcript
- [Beret Guy, holding his arms out, stands behind a large orange pumpkin with the stem on top. It is sitting on a table. The pumpkin has been carved out as a lamp with large hole, and a lit candle is visible in the hole. The hole is in the shape of another carved out pumpkin. An interviewer speaks from off panel.]
- Interviewer (off-panel): So what did you—
- Beret Guy: I carved a pumpkin!
- Interviewer (off-panel): ...
- [Black Hat stands behind a large orange pumpkin which has not been carved out as a lamp, but the stem at the top has been removed and is placed tilting on the side of the pumpkin. It is sitting on a table. A gray box with a labeled and a warning stands next to and partly in front of the pumpkin. On the end of the box there is a label at the top with unreadable text and below that some kind of drawing with a circle at the top. The interviewer speaks from off panel.]
- Interviewer (off-panel): Taking on teen vandals, I see.
- Black Hat: Heavens, No. My pumpkin simply has chest pains. In fact, I'll leave a note warning them not to smash it.
- Label:
- Nitro-
- glycerin
- Waning: Do not shake
- [Megan stands next to a large orange pumpkin with the stem on top. It is sitting on a table. The pumpkin has been carved out as a typical Halloween lamp. The bottom part of a white candle stick is visible in the mouth shaped hole. The hole is in the shape of a typical jack-o' lantern, with two slanted eyes, double slit nose and a smiling mouth with a tooth sticking out from both upper and lower lip, on either side of the candle stick.]
- Megan: My pumpkin's name is Harold. He just realized that all the time he used to spend daydreaming, he now spends worrying. He'll try to distract himself later with holiday traditions, but it won't work.
- [Cueball stands next to a two orange pumpkins with their stems on top, the left pumpkin is slightly larger than the right which is partly in front of the larger pumpkin. They have not been carved out even though a knife lies next to them to the right in front of Cueball on the table where they both stand. The interviewer speaks from off panel.]]
- Cueball: I carved and carved, and the next thing I knew I had two pumpkins.
- Interviewer (off-panel): I told you not to take the axiom of choice.
Discussion
I'm bringing over a comment from the blog, because it helps in understanding the Banach-Tarski theorem and Axiom of Choice.
- I realize that you probably aren’t checking these comments anymore, but I’m math grad student currently wasting time I shouldn’t be, and can’t resist answering this question.
- So the short answer is pretty much exactly what Jonathan said–the Axiom of Choice is in someways the “extra” axiom of ZFC set theory (in fact ZFC stands for “Zermelo–Fraenkel set theory *with the axiom of choice*”) and for a long period of time mathematicians were attempting to either
- (1) prove the axiom of choice as a consequence of the other axioms of set theory
- or
- (2) discover a compelling reason why we should not accept the axiom of choice.
- The Banach-Tarski theorem was published at the height of the debate/research, and is still frequently the first thing cited by someone who doesn’t accept the axiom of choice (although most working mathematicians I know do accept the axiom of choice, in part because it just seems silly to handicap yourself unnecessarily).
- What makes the Banach-Tarski theorem seem so paradoxical is simply the fact that they show it is possible to cut a ball into a finite number of pieces (5, to be specific) and reassemble these pieces only using rotations and translations (ie, only by movements you can make with your own hands) to produce two balls, each identical in volume to the first–ie, in someways "1 [ball] = 2 [balls]", which certainly feels a bit shady.
- All of the other theorems and axioms on which the Banach-Tarski theorem relies are standard and relatively accepted areas of mathematics, while the axiom of choice (which is used at a key point of the proof to “choose” elements not fixed in place by particular nice rotations of the sphere) was not, so many people consider this a reason not to accept the axiom of choice.
- If we don’t accept the axiom of choice, it becomes impossible to pick those points, and the whole proof breaks down. In fact, it can also be shown that if we don’t accept the axiom of choice, there don’t exist any “unmeasurable” sets–sets such as those created in the cutting of the ball whose volume we can’t really talk about, as it would need to be add up to 1 (after all those 5 sets together form a ball of volume 1!) and add up to 2 (after all, those 5 sets together form two balls of volume 2!), which makes some people very happy with the idea of rejecting the axiom of choice.
- For myself, I actually *don’t* find the theorem very paradoxical at all–these 5 “pieces” into which the ball is decomposed are incredibly crazy, and nothing you could ever cut with a scalpel, or even a laser, no matter how good you are. Additionally, the theorem really follows relatively easily from a theorem everyone accepts, which is that you can similarly split up the group of rotations to four disjoint pieces and “reassemble” them via rotations into two copies of the group of rotations. It seems a little odd at first, but the thing to keep in mind that any time infinite things get involved, things are going to get a little odd (after all–what’s infinity + infinity? What about infinity/2?). Honestly, at this point, the thing which I find the most “paradoxical” about the Banach-Tarski paradox is that it can’t be done by dividing the ball into only 4 pieces, but this could be a sign I’ve been drinking the math-koolaid for too long. — Ashley
- To clarify something on the point above, the 5 "pieces" are described as sets of points rather than actual objects with areas, and thus cannot be created in physical space. I edited the page to accentuate this, and to remove what I believed to be a contradictory statement. The original statement "This paradox has been proven for just about anything... except objects made of atoms, which our universe is comprised of." implies that a) objects made of atoms are not considered divisible and b) that most things are considered divisible. "Just about anything" could mean the physical universe, in which case the truth is that nothing is divisible and "just about anything" is misguiding, or both things that are within the physical universe and hypothetical things, in which case it deserves further explanation. Thus I edited to explain slightly further, being a safe move to improve the article in the case of either intention.108.162.215.72 05:17, 18 May 2014 (UTC)
- "In fact, it can also be shown that if we don’t accept the axiom of choice, there don’t exist any “unmeasurable” sets"
- That's not right. If we don't accept the axiom of choice, we can neither prove nor disprove the existence of unmeasurable sets. If we could disprove the existence of unmeasurable sets without the axiom of choice, we could disprove it with the axiom of choice by just not bothering to use it. 108.162.216.58 03:59, 25 October 2014 (UTC)
There you have it. lcarsos (talk) 17:42, 4 September 2012 (UTC)
"sited" changed to "cited"--DrMath 08:52, 4 June 2014 (UTC)
- There are so many *citation needed* options here, but I don't know how to put them in :( 162.158.78.82 23:33, 1 May 2017 (UTC)
- http://www.explainxkcd.com/wiki/index.php?title=285:_Wikipedian_Protester&action=edit§ion=3 Two opening-braces, "Citation needed", two closing-braces.[citation needed] 108.162.216.106 22:51, 2 July 2017 (UTC)
