Difference between revisions of "1310: Goldbach Conjectures"
BlueMoonlet (talk | contribs) (→Explanation: Many ways to think of counter-examples) |
BlueMoonlet (talk | contribs) (→Explanation: Improve explanation) |
||
| Line 12: | Line 12: | ||
{{w|Goldbach's conjecture}}, {{w|Goldbach's weak conjecture}}, and the {{w|Twin prime|twin prime conjecture}} are unsolved problems in mathematics relating to {{w|prime numbers}} (numbers whose only {{w|divisors}} are 1 and itself). | {{w|Goldbach's conjecture}}, {{w|Goldbach's weak conjecture}}, and the {{w|Twin prime|twin prime conjecture}} are unsolved problems in mathematics relating to {{w|prime numbers}} (numbers whose only {{w|divisors}} are 1 and itself). | ||
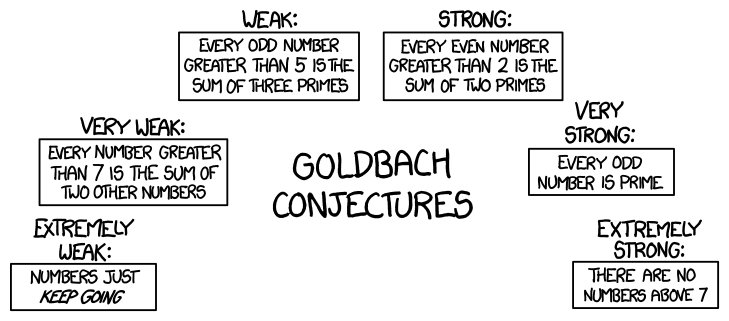
| − | + | Randall is riffing on the relationship between "strong" and "weak" logical statements, which are an interplay between the boldness or usefulness of a statement and the ease with which it might be proven to be true. For example, if Goldbach's conjecture (given in the comic under the label "strong") could be proven to be true, it would automatically imply that Goldbach's weak conjecture (given in the comic under the label "weak") is also true, because any odd number greater than 5 can be expressed as 3 (a prime number) plus an even number greater than 2 (which, per the strong conjecture, would itself be the sum of two prime numbers), resulting in a way to express the original odd number as the sum of three prime numbers. The weak conjecture does not, however, imply the strong conjecture. Goldbach's weak conjecture has been claimed to have been proven true, while Goldbach's strong conjecture remains unsolved. | |
| − | Goldbach's weak | + | This comic plays on the "strong" and "weak" naming of Goldbach's conjectures by extending it to further degrees of strength or weakness. The "very weak" and "extremely weak" conjectures are indeed implied by Goldbach's weak conjecture, just as the weak conjecture is implied by the strong one. The "very strong" and "extremely strong" conjectures are extensions of Goldbach's strong conjecture, even as it is an extension of the weak conjecture. However, the "very weak" and "extremely weak" conjectures are so obviously true that they are hardly worth stating, while the "very strong" and "extremely strong" conjectures make such bold claims that they are obviously false. |
| − | The title text refers to the twin prime conjecture, which states that there are an infinite number of pairs of primes that differ by 2. | + | The title text refers to the twin prime conjecture, which states that there are an infinite number of pairs of primes that differ by 2, and then applies the same spectrum of "weak" and "strong" statements to it. |
[[Randall]]'s weak twin prime conjecture states that there are an infinite number of pairs of primes. This is clearly true. Per {{w|Euclid's theorem}}, there are an infinite number of primes. Unlike the actual twin prime conjecture (which specifies a distance of two), this conjecture does not specify a required distance. Thus, any pair from the infinite set of primes suffices. An example is 5 and 13. | [[Randall]]'s weak twin prime conjecture states that there are an infinite number of pairs of primes. This is clearly true. Per {{w|Euclid's theorem}}, there are an infinite number of primes. Unlike the actual twin prime conjecture (which specifies a distance of two), this conjecture does not specify a required distance. Thus, any pair from the infinite set of primes suffices. An example is 5 and 13. | ||
| Line 22: | Line 22: | ||
His strong twin prime conjecture states that every prime is 2 less than another prime. There are many ways to think of counter-examples to this statement, which is obviously false (thus Randall's humorous {{w|hedge (linguistics)|hedge}} that some prime numbers "may not look prime at first"). | His strong twin prime conjecture states that every prime is 2 less than another prime. There are many ways to think of counter-examples to this statement, which is obviously false (thus Randall's humorous {{w|hedge (linguistics)|hedge}} that some prime numbers "may not look prime at first"). | ||
| − | The tautological prime conjecture states that it itself is true, while making no statement about primes. It is not | + | The tautological prime conjecture states that it itself is true, while making no statement about primes. It is not technically a tautology but more of a plain assertion. Randall has mentioned tautologies before in [[703: Honor Societies]]. |
==Transcript== | ==Transcript== | ||
Revision as of 18:32, 30 December 2013
Explanation
| |
This explanation may be incomplete or incorrect: surely not quite complete yet... If you can address this issue, please edit the page! Thanks. |
Randall is riffing on the relationship between "strong" and "weak" logical statements, which are an interplay between the boldness or usefulness of a statement and the ease with which it might be proven to be true. For example, if Goldbach's conjecture (given in the comic under the label "strong") could be proven to be true, it would automatically imply that Goldbach's weak conjecture (given in the comic under the label "weak") is also true, because any odd number greater than 5 can be expressed as 3 (a prime number) plus an even number greater than 2 (which, per the strong conjecture, would itself be the sum of two prime numbers), resulting in a way to express the original odd number as the sum of three prime numbers. The weak conjecture does not, however, imply the strong conjecture. Goldbach's weak conjecture has been claimed to have been proven true, while Goldbach's strong conjecture remains unsolved.
This comic plays on the "strong" and "weak" naming of Goldbach's conjectures by extending it to further degrees of strength or weakness. The "very weak" and "extremely weak" conjectures are indeed implied by Goldbach's weak conjecture, just as the weak conjecture is implied by the strong one. The "very strong" and "extremely strong" conjectures are extensions of Goldbach's strong conjecture, even as it is an extension of the weak conjecture. However, the "very weak" and "extremely weak" conjectures are so obviously true that they are hardly worth stating, while the "very strong" and "extremely strong" conjectures make such bold claims that they are obviously false.
The title text refers to the twin prime conjecture, which states that there are an infinite number of pairs of primes that differ by 2, and then applies the same spectrum of "weak" and "strong" statements to it.
Randall's weak twin prime conjecture states that there are an infinite number of pairs of primes. This is clearly true. Per Euclid's theorem, there are an infinite number of primes. Unlike the actual twin prime conjecture (which specifies a distance of two), this conjecture does not specify a required distance. Thus, any pair from the infinite set of primes suffices. An example is 5 and 13.
His strong twin prime conjecture states that every prime is 2 less than another prime. There are many ways to think of counter-examples to this statement, which is obviously false (thus Randall's humorous hedge that some prime numbers "may not look prime at first").
The tautological prime conjecture states that it itself is true, while making no statement about primes. It is not technically a tautology but more of a plain assertion. Randall has mentioned tautologies before in 703: Honor Societies.
Transcript
- Goldbach Conjectures
- Weak
- Every odd number greater than 5 is the sum of three primes
- Strong
- Every even number greater than 2 is the sum of two primes
- Very weak
- Every number greater than 7 is the sum of two other numbers
- Very strong
- Every odd number is prime
- Extremely weak
- Numbers just keep going
- Extremely strong
- There are no numbers above 7
Discussion
If a bot can create the text I read here, we have made great strides in artificial intelligence. Probably a human editor forgot to change the "incomplete/incorrect" heading. Tenrek (talk) 05:53, 30 December 2013 (UTC)
- Yes, I'm a bot. 199.27.128.62 21:42, 30 December 2013 (UTC)
I thought that {{incomplete|Created by a BOT}} means that the template was inserted by a BOT. 173.245.50.84 13:55, 30 December 2013 (UTC)
- It does mean that. But as others edit the page, they should keep the "incomplete" reason up-to-date. I've changed it to "incomplete|surely not quite complete yet..." ;) Nealmcb (talk) 14:28, 30 December 2013 (UTC)
It all seems to work except that the extremely strong seems to imply the opposite of the extremely weak Djbrasier (talk) 02:19, 31 December 2013 (UTC)
- I think the mistake is in the implication of the very weak to the extremely weak version. In fact, if there is any connection between those two statements it is an implication that goes the other way round. If the extremely strong version is true, we are not looking at the natural numbers. Thus, "Every number greater than 7 is the sum of two other numbers." does not imply "Numbers just keep going.", at all. (Also this accounts for no numbers at all, so the very weak version would still be correct.) Then there is the case that the extremely strong version is false. An implication from something false to anything is always true. --173.245.53.200 07:30, 1 January 2014 (UTC)
---I disagree with this, as it is not incorrect to say that "numbers keep going towards seven" as there are an infinite number of numbers approaching 7. Also, the extremely weak conjecture could easily refer to numbers in the negative direction only. 173.245.54.61 (talk) (please sign your comments with ~~~~)
I always find it amusing that people assume that something phrased 'scientifically' is therefore right, whereas something phrased unscientifically (eg religious beliefs taken on faith) are automatically wrong. There seems to be an unexamined assumption that science is some magical dark art for uncovering infallible truths. Of course science is really just a methodological system for testing theories. Whenever I try to explain this concept, I try to come up with a general, untestable (non-scientific) assertion that is nonetheless true, alongside a very specific, repeatedly testable (falsifiable) assertion that is therefore eminently scientific, but which happens to be wrong. (Eg "it sometimes rains on Wednesday" and "it rains at least 100mm every Wednesday in Riyadh"). So for me this comic is a commentary on that principle - that the "strength" of a statement is only really impressive if it has also survived testing. Tarkov (talk) 10:47, 31 December 2013 (UTC)
- The assumption is not "that science is some magical dark art for uncovering infallible truths" but that science works. Bitches. Also, the example you have given is quite bad considering that your first statement is so vague that it is essentially meaningless and apparently, what you want to say with your second statement is that falsifiable claims are falsifiable, which is pretty trivial. Finally, the statements that are phrased unscientifically are not assumed to be automatically wrong but they are impossible to be proven or disproven and are often worded so vaguely that nobody in the known universe knows just what the hell they are supposed to even mean. They are just empty phrases that carry no information whatsoever. --173.245.53.200 07:30, 1 January 2014 (UTC)
According to the strong twin prime conjecture, all positive numbers greater than one are prime, due to 2 and 3 both being prime and extrapolation on primes from there. Thus, this nearly proves the very strong Goldbach conjecture, excluding one. Should this be noted in the explanation? 108.162.237.4 02:08, 1 January 2014 (UTC)(Kyt)
- I don't know if it's worth complicating things to bring the matter up. It's potentially more complicated than a simple error; in Goldbach's day, people still sometimes thought of 1 as a prime number (which simplifies his conjectures). —TobyBartels (talk) 18:00, 1 January 2014 (UTC)
This also reminds me of those psychological tests that ask how you feel about this and that. 108.162.226.228 15:02, 1 January 2014 (UTC)
Don't forget the first rule of tautology club. --141.101.98.236 18:07, 1 January 2014 (UTC)
- Moved from explain
I disagree with this, as it is not incorrect to say that "numbers keep going towards seven" as there are an infinite number of numbers approaching 7. Also, the extremely weak conjecture could easily refer to numbers in the negative direction only. (Edited by some people.) --Dgbrt (talk) 18:18, 10 January 2014 (UTC)
"Therefore, the "extremely strong" conjecture could not possibly imply (however indirectly) the validity of the "extremely weak" conjecture, as it would if proved true."
- It can be argued that since the "extremely strong" conjecture is obviously a contradiction (as in the logical sense, "a formula that's always false"), thereby, can imply any other formula. That is, if p is always false, then (p->q) for any q is always true. In this sense, if the "strong" version gets proved somehow, you get an inconsistent logical system, in which each and every formula can be proved as true, including those weaker forms. 108.162.215.56 13:03, 9 February 2014 (UTC)
This sentence is problematic: "The weak conjecture does not, however, imply the strong conjecture." "A does not imply B" technically means "A and not B" which, I'm sure, isn't what was meant. I added "in any evident way" which I think corrects it. 199.27.133.59 08:52, 10 July 2014 (UTC)
The paradoxical prime conjecture states that the paradoxical prime conjecture is false. --108.162.250.220 07:58, 12 October 2014 (UTC)
KYT's conjecture - prime numbers pattern
KYT's conjecture is described as follows:
a is a positive integer and is even, a>=8, b=a+18, a=c+D, c, D,E are prime numbers.
a=c+D b=c+E
E=D+18=b-c
There must be a prime number c that satisfies the two equations above. More examples are as follows:
10=3+7;5+5 28=5+23;11+17 46=3+43;5+41;17+29;23+23 64=3+61;5+59;11+53 ;17+47;23+41 82=3+79;11+71 ;23+59;29+53;41+41
Hello, I am not a regular user here (I have no account), but I am a working analytic number theorist. Harald Helfgott's proof of the ternary (i.e., weak) Goldbach conjecture has indeed been accepted as correct.
162.158.126.204 12:26, 27 August 2024 (UTC) Frank Thorne