113: Riemann-Zeta
| Riemann-Zeta |
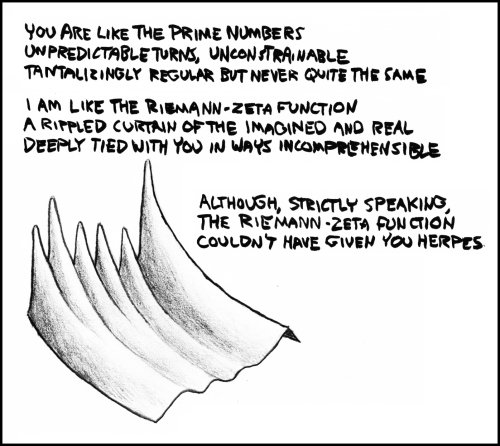 Title text: The graph is of the magnitude of the function with the real value between 0 and 2 and the imaginary between about 35 and 40. I've misplaced the exact parameters I used. |
Explanation
A prime number is any positive whole number greater than 1 that is only divisible by itself and 1. There is an infinite number of prime numbers, but they are somewhat elusive since there is no known function that yields all prime numbers and only prime numbers. The Riemann zeta function is an infinite series of 1/n^s where s is a complex number (i.e. s = x + i where x is real and i is imaginary) and is summed from n=1 to infinity. As the image text indicates, the graph in the picture is of the Riemann-Zeta function in the complex plane. Leonhard Euler proved that there is a relationship between the Riemann-Zeta function and prime numbers, which explains Randall's statement of the deep ties between the two.
Here Randall appears to be talking to his significant other, comparing her to prime numbers and himself to the Riemann-Zeta function. It is mathematically correct and quite poetic, until he mentions that his relationship differs from the comparison because "The Riemann-Zeta function couldn't have given you herpes." Which is quintessentially Randall.
Transcript
- [A z=fn(x, y) plot, with pointy spikes on the back sloping to a relatively flat front.]
- You are like the prime numbers. Unpredictable turns, unconstrainable. Tantalizingly regular but never quite the same.
- I am like the Riemann-zeta function. A rippled curtain of the imagined and real. Deeply tied with you in ways incomprehensible.
- Although, strictly speaking, The Riemann-zeta function couldn't have given your herpes.
Discussion
[looking at the dearth of comments] Math is weird. -Kazvorpal (talk) 22:28, 19 September 2019 (UTC)
Wow, no one has anything to say here... 108.162.245.225 19:27, 19 November 2023 (UTC)
