1856: Existence Proof
| Existence Proof |
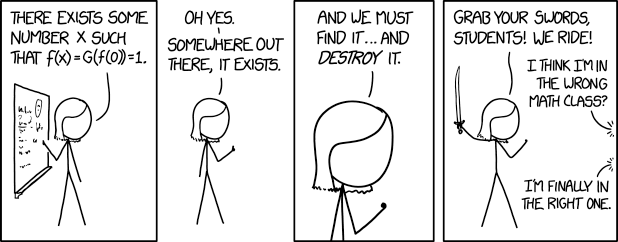 Title text: Real analysis is way realer than I expected. |
Explanation
| |
This explanation may be incomplete or incorrect: Needs more work, especially an explanation of the G() function. If you can address this issue, please edit the page! Thanks. |
This seems to be a continuation of 982: Set Theory, where numbers were "executed" to prove a point. This time it goes even further, with Miss Lenhart (and students) taking up arms to destroy the function value, which they have proven to exist. In the last panel, some students off screen begin to wonder if they are in the right class, as normal math classes do not take up swords to fight abstract concepts.[citation needed] Another student remarks that they are finally in the right math class, implying that this is the kind of thing they wanted from their math curriculum all along.
The phrase "We ride" is commonly used in rallying battle cries, particularly in fantasy or medieval dramas where characters are preparing to enter combat on horseback. Variations of the phrase are used several times in The Lord of the Rings, for example. In addition, the number which must be destroyed could be a reference to the One Ring.
The title text refers to Real Analysis, a branch of mathematics dealing with real numbers and real-valued functions (as opposed to studies dealing with imaginary numbers in the complex plane). As the speaker implies, Real Analysis is supposed to remain confined to the theoretical realm of mathematics; certainly nobody signing up for such a class would ever expect to be embroiled in a crusade against intangible constructs! Taken out of its mathematical context, "analysis" literally means "breaking down", referring to the teacher's intention to cut things up with a sword. The use of the neologism "realer" conveys that the situation has suddenly developed unusually high stakes, in a manner similar to the phrase "shit just got real". This nuance would be lost if the word "realer" were replaced with the technically correct phrasing of "more real".
Transcript
- [Miss Lenhart stands in front of a whiteboard and points at calculations written on it.]
- Miss Lenhart: There exists some number x such that f(x)=G(f(0))=1.
- [Miss Lenhart moves her arm in a frameless panel.]
- Miss Lenhart: Oh yes.
- Miss Lenhart: Somewhere out there, it exists.
- [Zoom-in on Miss Lenhart raising a fist.]
- Miss Lenhart: And we must find it... and destroy it.
- [Miss Lenhart raises a sword.]
- Miss Lenhart: Grab your swords, students! We ride!
- Student #1 (off-screen): I think I'm in the wrong math class?
- Student #2 (off-screen): I'm finally in the right one.
Discussion
Whoever added the citation needed got more of a laugh out of me then Randall did this morning. Well done. --172.68.142.29 17:32, 28 June 2017 (UTC)
- I hope you enjoy the joke just as much the second time. And the third. And the fourth. And the fifth. And the sixth. And the... 162.158.75.100 00:56, 29 June 2017 (UTC)
- [citation needed][citation needed][citation needed][citation needed][citation needed][citation needed][citation needed] 162.158.78.28 03:54, 2 July 2017 (UTC)
- I don't re-read old pages of explain xkcd so often it would stop being funny. -- Hkmaly (talk) 04:11, 29 June 2017 (UTC)
- I disagree, I must have seen 5 or 6 crazy "citation needed"s in recent memory, and for me it never stops being funny. :) A couple of faves have been that a baby could not plan and execute a jewel heist [citation needed] and 5 million years is longer than the average lifespan [citation needed]. :) I might have to start collecting these. NiceGuy1 (talk) 04:22, 30 June 2017 (UTC)
- <AOL>Me too!</AOL> RoyT (talk) 07:10, 29 June 2017 (UTC)
- [ Citation needed ]Mathmannix (talk) 16:23, 18 July 2017 (UTC)
Does the function have any special hidden meaning, or is it just some random function? Thawn (talk) 20:23, 28 June 2017 (UTC)
- Yeah - I wondered that too. But I'm not sure if there is enough information to know. SteveBaker (talk) 21:28, 28 June 2017 (UTC)
- Without knowing what the functions are, there's no way to tell. Gmcgath (talk) 23:52, 28 June 2017 (UTC)
- Unless I'm way off-base, There are an infinite number of solutions. For example, let's assume f(x)=2x and G(x)=x+1. X can, in this example, be literally any number because G(f(0)) = G(2*0) = G(0) = 0+1 = 1. As long as G(x) takes the result of f(0) and makes it equal to 1, it doesn't matter what f(x) is. 162.158.62.225 13:25, 29 June 2017 (UTC)
- Well, f(x) would also have to be equal to 1, not just G(f(0)). 108.162.237.154 14:14, 5 July 2017 (UTC)
- They're defined to be equal. It's right there on the board! Nitpicking (talk) 01:37, 29 December 2022 (UTC)
- Mitchell Feigenbaum's study of the universality of period-doubling ratios involved a function that solved f(0)=1 and af(x)=f(x/a) (IIRC). The equation in the comic reminded me of this, though it's not quite right. 172.68.78.58 16:55, 29 June 2017 (UTC)
"I'm finally in the right one," made me laugh more than usual. It added character to Offscreen Student #2, something that the comic usually lacks HisHighestMinion (talk) 03:56, 29 June 2017 (UTC)
- Could be Black Hat? Observer of the Absurd (talk) 12:23, 6 May 2019 (UTC)
According to https://en.wikipedia.org/wiki/Constructive_proof "existence proof" means a non-constructive proof. Such proofs are annoying to some mathematicians as they claim existence of something but do not show how to find it. So I fully understand the teacher that she wants to grab a sword and finally find it. 162.158.202.76 08:54, 29 June 2017 (UTC)
Jokes on her. The number is a | nonstandard integer. 172.68.78.28 10:12, 29 June 2017 (UTC)
To me, the comic reads (especially with the title text) with the implication that the teacher is encouraging the students to help her actually fight real numbers in real life, as if the platonic idea of numbers was "realer" than we think. 172.68.215.98 10:42, 29 June 2017 (UTC)
The sentence "There exists some number x such that f(x)=G(f(0))=1." boils down to "There is an x such that f(x)=1". The part with G(f(0)) is only a way to arrive at 1. For some reason there is an x that satisfies f(x)=G(f(0)), and since G(f(0))=1, it is equivalent to f(x)=1. 141.101.76.142 (talk) (please sign your comments with ~~~~)
