1347: t Distribution
| t Distribution |
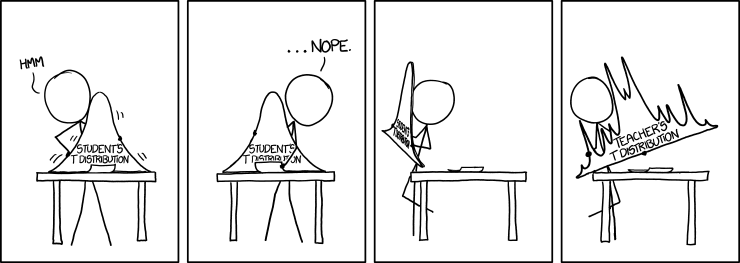 Title text: If data fails the Teacher's t test, you can just force it to take the test again until it passes. |
Explanation[edit]
The Student's t-distribution is a class of probability distribution used in statistics to model small sample sizes. "Student" was the pseudonym of William Sealy Gosset, an employee of Guinness Brewery who discovered it.
A Student's t distribution is similar to a normal symmetric bell curve distribution, but has "fatter tails"; thus, the one shown in the comic is roughly the right shape. A "Teacher's" t-distribution is a joke (pun) made up by Randall.
The comic is a play on the name "Student", the pseudonym of the creator, versus the "Teacher". The idea is that a "teacher's" distribution would be more complex, and that it would be used for fitting data when the student's distribution wasn't sophisticated enough. Of course, in actuality, such a complex distribution as the one shown in the comic would have many parameters, and in practice would probably lead to overfitting and/or bias. Thus, the comic (and the title text) can be seen as making fun of the idea that more complex is always better, or perhaps of the idea that a statistician's job is to use more and more sophisticated tools to force the data to yield a "publishable" result, rather than to use the simplest appropriate tool and let the chips fall where they may.
Cueball tries to "fit" a distribution to the data on the paper. This is the usual jargon for when a statistician is trying to model their data as coming from some underlying probability distribution, and the comic makes a pun with the physical meaning of "fit". In the second panel, Cueball decides that the Student's T distribution does not fit his data well (the data failed the Student t-test), and decides to pull out the more complex Teachers t-distribution instead (the teachers t-test - which the data is not allowed to continue to fail). Note that "test" is what statisticians do to data to see if it fits some distribution, but it is also another word for "examination."
The Students t distribution relates the average of a small sample to the "true" population average, under the assumptions, unobjectionable in many contexts, that there is such a "true" value, and that the samples are independent and normally distributed with equal variance. As such, unless the data on Cueball's paper contain many small groups which radically violate these assumptions somehow, there is no way Cueball's data could falsify the t distribution. In particular, a single number (for the average of one group) or a small set of numbers (for the averages of several numbers) will never make a nice smooth curve, but an average statistician would see that as normal statistical noise that would even out over time, not as a reason to prefer a complex, spiky curve such as the supposed "teacher's" distribution. But of course, Cueball's access to a secret, cooler-looking distribution makes them more badass than a mere average statistician... or does it?
Ironically, the Teacher's T Distribution shows equal variance, itself proving the appropriateness of the Student's T Distribution.
The title text plays on the word "test". The first part of the sentence refers to a potential "Teacher t-test" which would be used in a statistical context to test for the significance of some observation, as opposed to the real "Student's t-test" which is used to determine if two sets of data differ by a statistically significant amount. On the other hand, the second part of the sentence refers to the possibility for students to take tests (or exams) until they pass - or to teachers who forces students to take the test again and again until they pass. The resulting sentence may refer to statistical fallacy, or the (conscious or unconscious) action of manipulating observations or misconducting experiments to give statistical significance to a false fact.
Transcript[edit]
- [A physical bell-curve-shaped object labeled "Student's t distribution" is resting on a table. Cueball is working with it and a piece of paper.]
- Cueball: hmm
- [Cueball looks at the piece of paper.]
- Cueball: ...nope.
- [Cueball picks up the object and begins to walk off the panel with it.]
- [Cueball comes back onto the panel, now carrying an object shaped like a much more complex curve, with many symmetric spikes and dips, labeled "Teacher's t distribution".]
Discussion
http://en.m.wikipedia.org/wiki/Student%27s_t-test
173.245.50.73 05:20, 26 March 2014 (UTC)Adam
As a layman, I still have no idea what the comic's about. Is it possible to clear it up a lot more? LogicalOxymoron (talk) 05:37, 28 March 2014 (UTC)
I think this is a comment of the quality of education today - it is difficult to grade students on a distribution curve and even more so when you take into account the distribution curve of the teachers ability. 108.162.249.205 (talk) (please sign your comments with ~~~~)
- I thought this as well, my interpretation of the comic was Cueball attempting to fit the data with a "Student t-distribution", realizing that the t-distribution poorly fit, and so replaced it with a "Teacher t-distribution" which has a stronger correlation with the data on the piece of paper presumably; the data in question concerning the scholastic success of students. This comic in part seemed to be poking fun at scientists misappropriating the causation of a recognized phenomena. Like the basic statistics example of people finding a correlation in children between tooth decay and vocabulary when, surprise surprise, both tooth decay and vocabulary are strongly correlated with age. 108.162.249.214 (talk) (please sign your comments with ~~~~)
I noticed the teacher's curve is symmetrical, and after further inspection it could be interpreted as an edge detection: high values show where an edge occurs. The two highest peaks would nicely align with the edges of the paper, the next highest peaks fit the edges of the table, and the rest could be approximation artefacts, as they're equidistant and rather insignificant compared to those four. I'm not statistics pro, but maybe that rings someone's bells? 108.162.210.239 07:56, 26 March 2014 (UTC)
That's the T. No shade. 172.68.132.151 07:03, 14 May 2021 (UTC)
- Interesting observation. It may play into an age-long legend told and re-told among the students that some teachers grade papers by tossing the whole pile in the air; those sheets that land on the teacher's desk get a pass, those falling to the floor get a fail. Sometimes the story gets modified in such a way that papers falling on the teacher's book (or other object) laying on the desk will get a higher marking than those simply hitting the desk. The latter version would explain the higher sheet-size-apart peaks. 108.162.210.111 08:57, 26 March 2014 (UTC)
To be more explicit, I think the sheet of paper represents some data. Cueball is not happy with the results of applying Student's t test, so ze is trying more complex tools in the hope of getting significance. -- TimMc / 173.245.52.27 11:51, 26 March 2014 (UTC)
- I would upvote this comment if allowed. As an aside, there are some teachers who think a class' grades will always fall into a nice t Distribution (thus the expression "grading on a curve") and others who vehemently hate the notion. Source: my 3-year stint as a math teacher in an urban high school. Smperron (talk) 14:06, 26 March 2014 (UTC)
Man, normally these explanations clear the comic right up for me, but I've read this one thrice now and I still can't figure out what a t-distribution is, much less a joke based on one. The only definition being a Wikipedia quote written in legalese doesn't help. So a t-distribution estimates...the probability of a population's average when there's unknown information?108.162.216.48 12:17, 26 March 2014 (UTC)
- The unknown information is the sample size (class size, for example) and standard distribution (by how much, on average, is something going to vary from the mean). The unknown information is not "in the data".Jarod997 (talk) 12:28, 26 March 2014 (UTC)
- Basically, if you have an underlying process that would produce samples with a Gaussian distribution with mean of 0, and stddev of 1, and then you pull a finite number of samples out of it, and do the usual "average" operation on those samples (i.e. sum them and divide by the number of samples) you would expect that that computed average would be close to zero. But it might not be! By chance the samples you pulled might mostly have been from the far right or left side of distribution and the average you got would be way off. Student's T distribution (for a certain number of samples, n) is basically "given that the underlying process a Gaussian with mean zero and stddev of 1, if I repeatedly take n samples from that distribution and compute the average of those samples to get an "estimated mean", this is how I expect that estimated mean to be distributed". Naturally, this is important in questions like "I took 100 samples and got an average of 0.02 -- does this mean that it is sensible to think that the mean of the underlying distribution is actually zero?"
- Of course, most of the joke is that the distribution is named "Student's", which is not strongly dependent on the nature of the statistics. Vyzen (talk) 12:42, 26 March 2014 (UTC)
- Okay, it's pretty clear to me now what the Student's t distribution is. I'm still not sure about the punchline though, how does the "Teacher's" t distribution come into play? Does the uneven distribution represent any phenomena in the academic world? Like, as suggested above, is this a joke about grading? 173.245.53.137 15:05, 26 March 2014 (UTC)
- Other than the symmetry, I'd almost suggest that the distribution could be real test scores. Typically tests will have a small number of questions worth multiple points and the scores might spike around levels that represent integral numbers of questions done perfectly, with the spaces in-between filled in by part marks. The teacher may have a bias towards giving perfect or zero scores per question. Vyzen (talk) 18:53, 26 March 2014 (UTC)
- Okay, it's pretty clear to me now what the Student's t distribution is. I'm still not sure about the punchline though, how does the "Teacher's" t distribution come into play? Does the uneven distribution represent any phenomena in the academic world? Like, as suggested above, is this a joke about grading? 173.245.53.137 15:05, 26 March 2014 (UTC)
The teacher's t-distribution looks like multiple spikier curves with different centres added together and it doesn't fit the table. Wwt (talk) 13:17, 26 March 2014 (UTC)
I took from it that the Students Distribution was too perfect, and real data would rarely yield those idealized results in a small sample size. That the teacher's distribution used actual numbers, with the occasional spikes. I took from the title text, the tendency of students, or anyone with pre-conceived notions, to keep redoing the test until they get the results they expect, in this case, the textbook result. 173.245.55.71 13:25, 26 March 2014 (UTC)
Any thoughts on the piece of paper he's trying to pull out from beneath the Students' T-distribution? 108.162.219.66 14:10, 26 March 2014 (UTC)
- I don't think he he trying to pull the paper from out beneath the t-distribution. I think he is placing the distribution on top of the paper to see if the data on the paper matches the distribution. In panel 2, he looks at the paper and decides that, no, it doesn't, so then opts to use another distribution - the Teacher's t-distribution and see if that works. The comic may be hinting that the t-distribution in grading, etc (since students and teachers are explicitly listed) is flawed. --Dangerkeith3000 (talk) 15:10, 26 March 2014 (UTC)
I may be over-simplifying it, but the 'Teachers' T looks like a reference to the 'double-hump programmer' idea, converted into a T-distribution. The other ideas cover the general principle, but this looks like a specific example as well. 108.162.221.48 15:47, 26 March 2014 (UTC)
I don't think the explanation really explains what a T-distribution is at all. I know it's googleable, but the point of an explanation is you shouldn't have to look it up afterwards. I don't like how lately all of the scientific/maths comics seem to be given explanations laden with technical terms that don't actually clarify anything. --Mynotoar (talk) 17:57, 26 March 2014 (UTC)
I did a quick calculation using mspaint, and it appears that the Student's t-distribution in the first panel is roughly 5780 px^2 in size; at the same time the area of the "Teacher's t-distribution" in the last panel is approximately 8125 px^2 (or 140% of the Student's distribution). Thus, using the Teacher's t-distribution as Cueball is intent on doing "is both illegal and illegitimate" (illegitimate = no scientific basis for such a distribution; illegal = this it not even a distribution per se). If Cueball goes on and publishes his results based on such approach, they will not be recognized by the international scientific community (except perhaps by Russia, Syria and North Korea). We, readers, therefore express our deep concern over Cueball's methods. Stpasha (talk) 18:27, 26 March 2014 (UTC)
I believe the joke has to do with "fitting data to a distribution": In the first panel, Cueball is trying to adjust the Student's T distribution on top of the data, which could be a play on "fitting" the data to the distribution. Statistically speaking, fitting data to a distribution is often done to figure out how likely the data were to have occurred, under the assumption that the underlying data generating process follows a particular distribution (like the Student's T). It looks like Cueball first tries to fit his data to a Student's T, and is dissatisfied with the fit. He then tries a much more complicated distribution - which, I think is jokingly called a Teacher's distribution on the premise that something to do with teachers is more complicated than something to do with students. The joke is that data often don't fit a simple distribution like the Student's T... they are nuanced and complex, and their underlying data generating process was far more complex. Amoorthy (talk) 19:50, 26 March 2014 (UTC)
- By the way, this is related to and compatible with the explanation given by Dangerkeith3000 above.Amoorthy (talk) 20:26, 26 March 2014 (UTC)
The title test could be referring to the tests aspiring teachers have to take in the US to get their credentials. It's sort of like a Bar- except you may take it as many times as you wish until you pass. 199.27.128.77 (talk) (please sign your comments with ~~~~)
- I thought it referred to the practice that some US school systems have of allowing students to take a test (examination) repeatedly until they pass it. 199.27.128.85 06:38, 30 March 2014 (UTC)
I predict that the "Teacher's t-distribution" is the new Cow Tools, and those with actual skill in statistics will drive themselves crazy over it. See [1] for clarification. 199.27.130.168 21:23, 26 March 2014 (UTC)
Could it be pointed out that the middle of the Teacher's distribution resembles the Tower of Mordor ? Underscoring the role of the Teacher... 173.245.52.25 (talk) (please sign your comments with ~~~~)
The explain says that the student distribution works when both the sample and the population have the same variance. Isn't that wrong--doesn't the sample tend to have a larger variance than the population under usual/ideal conditions? (I'm assuming the student distribution is meant for usual/ideal conditions.) Sciepsilon (talk) 00:44, 27 March 2014 (UTC)
- I believe the true variance of a sample should be the same as the true variance of the population. Perhaps you are thinking of Bessel's correction - using "n-1" in the denominator of the formula for estimating sample variance, instead of "n". If so: While it's true that Bessel's correction makes our estimate of the sample variance larger than if we'd used "n", the reason is that using "n" would have created an estimate that was too small - or, otherwise put, biased toward zero. (The Wikipedia article on Bessel's correction has the best explanation I've seen for why this is true - http://en.wikipedia.org/wiki/Bessel's_correction#The_source_of_the_bias.) What's key here is that Bessel's correction is a technique to correct our estimates of variance - the true variance of a sample is really the same as in the population. Amoorthy (talk) 16:20, 27 March 2014 (UTC)
My initial take is that in comic the students' understanding of the correct distribution is being evaluated as a function of the teacher's ability. That a poorly educated student reflects the ability of the teacherExternalMonolog (talk) 12:20, 27 March 2014 (UTC)ExternalMonolog
- This is exactly what I read. I think people are going all sorts of ways with calculating the T distribution. Clearly you need to just look at the graph and say, "looks like we're saying the data matches to the teacher skill set much more than the presumed result set." Joke over. Move on folks. -- Sean timmons (talk) (please sign your comments with ~~~~)
- Is it just me or the Teacher's T-curve looks like Barad-dur to anyone else..? 173.245.62.84 (talk) (please sign your comments with ~~~~)
I teach college courses, and find that exam scores typically do not fit a t distribution, or any symmetrical distrubution. They more often show several groups, or what statisticians call "modes." This is a source of frustration for a lot of teachers. I saw this comic as showing how far from "normal" a real teacher's distribution is. It seems to be two superimposed periodic functions instead of a distribution. Or Barad-dur.--Spencer9 (talk) 17:40, 20 June 2015 (UTC)
When this comic came out, it put me in mind of this article: https://deedy.quora.com/Hacking-into-the-Indian-Education-System In which it is shown that the indian university entrance scores do not follow a nice smooth distribution, but instead something with spikes at certain key values like the minimum pass mark. 108.162.250.157 (talk) (please sign your comments with ~~~~)
The comic could have been inspired by cases where a teacher actually forces the entire class to retake the exam because the results distribution don't fit the curve they expected. Example[2]
