2812: Solar Panel Placement
| Solar Panel Placement |
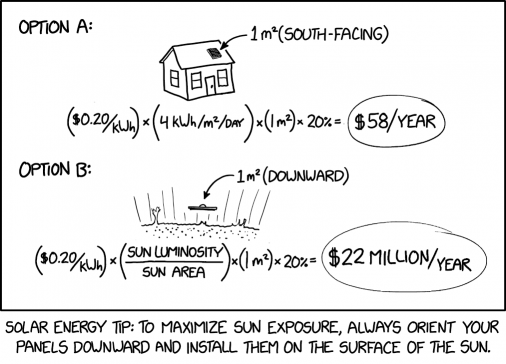 Title text: Getting the utility people to run transmission lines to Earth is expensive, but it will pay for itself in no time. |
Explanation[edit]
This is another one of Randall's Tips, this time a solar energy tip.
Solar panels generally produce electrical power in proportion to the intensity of sunlight striking them. In order to maximize energy production, it's generally recommended that panels be mounted at an angle that will receive the most light intensity, on average, and avoiding anything that might shade the panels. The comic might be poking fun at how sometimes solar panel arrays are installed in unusual orientations to ensure a south-facing orientation and pushing it to its logical extreme by assuming a panel on the sun.
Based on where the panel is located, the average amount of solar energy expected to strike it per day can be calculated (accounting for the angle of the sun, day and night cycles, and typical weather patterns). With this data, as well as the expected conversion efficiency and local cost of electricity, one can calculate the value of electricity the panel produces each year. In this case, Randall estimates the value of power produced by each square meter of solar cells at $58 per year.
The strip then proposes a rather intense comparison: place the identical solar panel downwards, on and towards the Sun, rather than upwards (upon a suitable equatorially-facing sloping roof), from the surface of the Earth. Due to the inverse-square law, this would result in much more solar energy striking the panel. If we assume that the solar cells could convert this energy to electricity at the same efficiency, then this would generate immense amounts of usable power, with the same calculation yielding $22 million per year as the value of a single panel in such a position.
Of course, such setup would clearly be impossible, for the simple reason that the panels would melt and then vaporize long before they reached the surface of the sun. In point of fact, current photovoltaics operate less effectively at higher temperatures, so even bringing them mildly closer to the sun would impair their efficiency, and eventually cause them to stop working altogether. This is in addition to the fact (acknowledged in the title text), that electricity produced at the sun's surface would be of little use to humans. The solution of "run[ning] transmission lines to earth" would obviously not be practical, even with millions of dollars at stake.
The assertion that the solar panel would pay for itself in no time seems flawed. For example the Helios 1 probe cost 260 million dollars in 1975 (approximately 1.5 billion dollars in 2023 money) and the Parker Solar Probe, which will fly 6.2 million km from the surface of the sun, also cost 1.5 billion dollars. The Parker Solar probe mass is 50kg, which is the same order of magnitude as a 1m² solar panel.
There are conceptual proposals, for siting solar arrays in space, but in orbit around earth, rather than than on the sun. This would allow for somewhat more solar intensity, and provide more consistent power, but the obstacles of launching the arrays into space and then transmitting the power remain serious pragmatic difficulties.
- Calculations
The formula Randall uses in this comic is electricity price × solar irradiance × panel area × panel efficiency = savings.
Electricity price is measured in dollars per kilowatt-hour, a unit commonly used by electric utility companies. In both cases, it is assumed to be $0.20 per kilowatt-hour, which is a reasonable estimate for domestic, retail electric rates in Randall's home of Massachusetts.
Solar irradiance, a special case of irradiance, is the total amount of power delivered to a surface by the Sun per unit area. This measurement varies substantially by geography, and must account for hours of daylight, angle of the sun, and weather patterns (all three of which vary by season). This number is expressed in kilowatt-hours per square meter per day, though this number is typically averaged for the whole year. Randall assumes a value of 4 kWh/m2/day, which is also reasonable for Massachusetts. He also calculates the value for the surface of the Sun as its total luminosity (electromagnetic power, ≈3.83×1026 W) divided by its total area (≈6.07×1018 m2), which comes out to around 6.31×107 W/m2 or 1.51×106 kWh/m2/day.
Solar panel area is measured in square meters.
Solar panel efficiency, a dimensionless quantity, is the fraction of solar power that a panel can effectively convert into electricity. Here, both panels are assumed to be 20% efficient, which is a reasonable estimate for commercially available photovoltaic cells operated near room temperature (300K).
Multiplying these quantities together yields a unit of money per unit time, per area (dollars per day per square meter with these specific units). For the parameters for the Earth-bound example, the formula yields $0.16 per day in effective earnings, which can be multiplied by 365 to get approximately $58 per year. For the parameters on the Sun, it instead yields $60,400 per day in earnings, or approximately $22 million per year.
It should be noted that these values are for each square meter of solar panel. Solar systems almost always consist of multiple panels. With the same assumptions, a 30-square-meter system (which is a relatively small, home system) would be worth $1,740 per year.
Transcript[edit]
| |
This transcript is incomplete. Please help editing it! Thanks. |
- [Heading:] Option A:
- [A stereotypical house with a single solar panel upon its roof and an arrow from a label:] 1 m2 (south-facing)
- [Formula:] ($0.20/kWh)×(4 kWh/m2/day)×(1 m2)×20% = $58/year
- [Heading:] Option B:
- [A short width of the Sun's undulating 'surface', with two solar prominances/flares and at their height (but above a different part of the surface) a solar panel with some attachment upon its upper surface, depicted horizontally aligned to the Sun and with an arrow from a label:] 1 m2 (downward)
- [Formula:] ($0.20/kWh)×(sun luminosity/sun area)×(1 m2)×20% = $22 million/year
- [Caption below the panel:]
- Solar energy tip: To maximize sun exposure, always orient your panels downward and install them on the surface of the sun.
Discussion
Can someone smarter than me do the math on running power lines to a panel on the sun? How long until it would pay for itself?172.70.214.125 05:08, 8 August 2023 (UTC)
- $22 million / $0.20 per kWH = 110 million kWH, divide by hours in a year and you get about 12549 kW. Google says the sun is 150 million KM away. IDK the exact details, but a calculator I found online suggests a copper cable with a cross-sectional area of 10m^2 can handle that amount of power transported 150 million KM. The density of copper's about 9 cm/g^3. 150 million km * 10 m^2 * 9 g/cm^3 = 1.35 * 10^16 kg. The cost to get one kilogram to Low-Earth Orbit according to google is >$10,000, let's just use that. Total cost to get to LEO: $1.35 * 10^20. Divide by profit per year => 6.1 trillion years. Tiln (talk) 07:10, 8 August 2023 (UTC)
- "The cost to get one kilogram to Low-Earth Orbit [...], let's just use that." Ummm... It's actually easier to leave the solar system than to rendezvous with Mercury (never mind attain even closer stability to the Sun's surface).
- I supose you could always go to an orbit a very long way away (near solar-escape) and add a little extra reverse delta-V to zero your orbital movement and then fall down. But you must not miss the pinprick Sun, or you're in a highly elliptic comet-like trajectory (with even higher demands needed to circularise at perihelion), so you need to be very precise about stopping and dodging through the gravity wells of any planets you plunge past. Not that not missing is going to do you much good, either.
- ...hmmm, hang on, maybe that's what the cables back to Earth are for. Spooled in/out just at the right rate (perhaps some bungee-chord included), it's how you stop just above the Sun's surface (at the limit of the conductive cable, then cut the retarding bungee just as you're stable enough at the bottom of the bounce!) and stay there. Ok, not a problem. It'll work after all[actual citation needed] and I withdraw all my petty objections! 172.71.242.82 10:43, 8 August 2023 (UTC)
- And then you'll have to deal with the end of the bungee cord retracting under it's own tension... and since it's no longer tethered to the craft it would probably whip back towards Earth. As an afterthought, have you ever been whipped by a released rubber band? Imagine that... but at a cosmic scale. I'd probably take my chances with the cables. Xkcdjerry (talk) 14:39, 8 August 2023 (UTC)
> "...IDK the exact details, but a calculator I found online suggests..."
- I don't disagree with >6 trillion year payback, but the cable-calculation is insufficient data. Say 1,000 Watts. This could be carried as 1 Volt 1,000 Amperes (roughly what comes out of a parallel panel) or as 1,000 Volts 1 Ampere. One is fat copper and hardly any insulation, the other is hair-fine copper and thick insulation. A proper design goes to the relative costs of conductor and insulation.
- Many on-Earth power lines rely on air for insulation and air can be really cheap. These favor extra high voltages. My neighbor has 20,000V; the long line in California is far over 200,000V, near 1MegaVolt. Space-vacuum is even cheaper, perhaps limited by tolerable size of the bushings at the earth station.
- 12,549 kW is like 13 MegaWatts. This could be carried as 13MV 1 Amp. Iron (for strength) #30AWG, 0.010" diameter, will carry 3 Amps. #30 is too fine for easy handling. #14 iron, "electric fence wire", is more manageable. About $100 per mile, I remember 93 million miles, so 9 Billion Bucks for wire. Plus deposit on the spools....... and shipping, and stringing.....
- BTW: 10m^2 may be a bogus extrapolation. Wires much over 0.25" 6mm 0.000,03m^2 suffer from internal heating. The cold of space helps, but there is no air convection cooling (no air, and no convection in zero-G).. a 3meter diameter cable won't carry 1% of what you would hope from small-wire experience.
- Yes, superconductors, hi-temp near the sun.... but this won't come until after the convenient Fusion Power which has been right around the corner literally since I was born.
--PRR (talk) 23:46, 8 August 2023 (UTC)
Congrats, you've just made the universe's smallest Dyson sphere component! 162.158.2.38 07:33, 8 August 2023 (UTC)
I'd just like to point out an error in Randall's math: The light incident on the panel would only be from the portion of the surface with line-of-sight to the panel. This fraction is called the "view factor", and has its own Wikipedia page, which I'm too lazy to link since I'm editing this on my phone. Carry on! 172.70.126.217 13:26, 8 August 2023 (UTC)
- I thought Sun luminosity (total outgoing light, in all directions from all points of itsvsurface) divided by Sun area (total luminescing surface area) multiplied by the 1m² (the actual 'capturable' parts) normalised to the effectively-in-contact portion of the Sun exactly in the way you're defining the view factor. (You could link to View factor by just trying
{{w|View factor}}, if you want, and edit it later if you're wrong.) - Further out, distance from the Sun factors in as an inverse square relationship, but it'll be negligable when you're practically at the Surface and close to the full 2pi steradian of incident light from a significantly greater area of emitting surface than the unit-area of receiving surface. Once you're at Earth-orbit distance, it's a tad below 70 nanosteradian of "panel view" and almost none of the light even from the directly facing square metre of Sun even comes close to the even smaller solid angle subtending the outwards spread of light.
- Set your panel at the height of various solar-surface features, you might not intercept much of the light (hence division by Sun's area) yet what you'll capture will be significant. Probably well beyond any long-(/medium-, perhaps even short-)term survivability of a lump of plastic, silicon and metals normally stuck on a house roof. 172.70.91.161 15:36, 8 August 2023 (UTC)
- I think view factor calculations are not needed here: Imagine the sun's surface covered with 1m² panels. Then all the outgoing light is captured by those panels. The incident light can be calculated by a simple area division. If the panels are further away, more panels are needed, and the incident light per panel is smaller (reverse square dependence on the distance from the center of the sun). (G.Rote) --172.69.150.109 05:31, 14 August 2023 (UTC)
Actually, the inverse square dependency yields results in the right ballbark: The Earth is 150 million km from the Sun's center, the Sun's surface is 700,000 km away from the center (radius of the Sun). The ratio is ~214. The squared ratio is ~46,000. The ratio between the monetary yields is $22 million/$58, which is around 380,000, bigger than 46,000. The additional loss factor of around 8.25 must come from the Earth's atmosphere and clouds, and the non-perpendicular angles during much of the day (and night). (My initial thought was that the ratio in earnings ought to be much bigger). (Günter Rote) --172.70.246.238 06:08, 14 August 2023 (UTC)
I was briefly confused because HVAC usually stands for heating, ventilation, and air conditioning, but is here used to mean high-voltage alternating current. 172.69.247.50 13:31, 8 August 2023 (UTC)
- I often have the opposite confusion... ;) 172.70.91.161 15:36, 8 August 2023 (UTC)
- Ah! Thank you!172.70.85.30 23:21, 8 August 2023 (UTC)
I ran some calculations on using rechargeable batteries to get power from the Sun to Earth, full markdown file is here is anyone to check it out.
TL;DR: it's way better than running powerlines to Earth but falls slightly shy of putting a panel on Earth, though that may be remedied by more precise data. Xkcdjerry (talk) 15:09, 8 August 2023 (UTC)
- New idea: maybe we can make some sort of belt in which one side has fully discharged batteries and the other has fully charged ones, so that gravity balanced out and we only need to do work against friction, that should raise the efficiency greatly, with some engineering we might get it above 0.1% or even 1%, however I don't have exact data on this so this remains the work of someone with more knowledge than me XD Xkcdjerry (talk) 15:38, 8 August 2023 (UTC)
The power line loss calculation is not at all valid. Lines on the surface of a planet lose far more power to heating and induction etc. In the cold vacuum of space, efficiency should be excellent. (This should also reference some of the https://what-if.xkcd.com/157/ problems though.) 172.69.65.206 18:21, 8 August 2023 (UTC)
- Given that standard transmission losses (for long distance HVAC power lines) are around 3% per 1000 km and the Sun is 150 million km away, the energy reaching the Earth would be 0.97^(150000), a truly negligible amount (10^-1985 of the input energy). In this case, you'd be better off literally sending huge packs of rechargeable batteries to Earth.
- ~~ Above comment not signed. ~~
- Aside from intentional absurdity, why run wires? Wireless power attenuation is very low in a near-vacuum; just beam the power back via microwave. That's how all the serious proposals work, & it seems both obvious & potentially quite practical... It's really just the survivability of anything parked so close to the sun's ejecta, that seems problematic, to me?
- ProphetZarquon (talk) 17:27, 9 August 2023 (UTC)
- Clearly the above is riffing directly off the "transmission lines" (i.e. strung physical cables) directly mentioned in the title text. Yes, melting/ablation/etc is going to be a major problem (as is sitting the panel directly above the so-called surface of the Sun, which seems to be different from putting it into Very Low Solar Orbit), but the fanciful issue of merely the cabling being a high initial capital cost is very much a Munrovian flight of fancy that's not particularly unusual in such punchlines.
- Yes, we'd do it differently 'IRL', but this is very much part of the whole treatment. For one thing, who puts just one 1m² PV panel on a rooftop, like that? (Never mind send it 1AU away from the owner's home.) Round here, the standard seems to be 14 panels (and non-square... 1m x 1.65m if my recent jotted measurements of those used upon a particular roof is not unrepresentative).
- It's a "spherical cow in a vacuum" sort of scenario, and we can choose to accept (or argue against) whatever bits of handwavium we feel like highlighting. So why not educate, inform and entertain? 172.70.86.7 21:16, 9 August 2023 (UTC)
I added an explanation of the two equations Randall used. Not sure how clear they are but I'm fairly certain the math checks out. DownGoer (talk) 05:53, 10 August 2023 (UTC)
I love you guys! I would be more concerned about keeping ym shiny new solar panel cooled enough to be efficient. That close to Earth, I would fear that my Solar Panel just melts, and that the solar wind woll blow the molten droplets all around. And I have yet to find an insurance company willing to cover any damaged property by this side effect of the fusion powered heat generator in our Solar System. Not worth the risk, IMHO. 172.71.182.45 16:45, 10 August 2023 (UTC)
- I would think they would reject your claim as resulting from an act of Sol.172.70.90.173 11:02, 14 August 2023 (UTC)
