881: Probability
| Probability |
 Title text: My normal approach is useless here, too. |
Explanation
| |
This explanation may be incomplete or incorrect: The difference between the two lines has to be explained. The "IV drip" is unknown to the most non native English speakers. If you can address this issue, please edit the page! Thanks. |
Randall wrote this comic after his fiancee was diagnosed with breast cancer. Two months after posting this strip, he posted this blog post explaining the cancer strips.
The title text could be a reference to the comic 55: Useless. Cueball's (and Randall's) normal approach — math — isn't much help in dealing with this emotional situation, either. The phrase "normal approach" may also in this case be a pun on the Normal distribution, which is another probability distribution that is commonly used in statistics. Interpreted this way, the title text states that the "Normal distribution" is not used as a hazard function.
Transcript
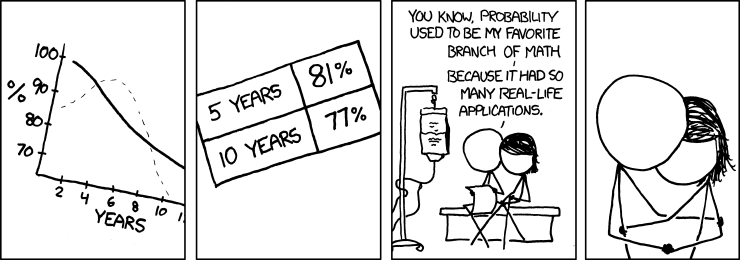
- [A plot of percent vs. years, with a solid and a dashed line. The solid line starts at 100%, and drops constantly. The dashed line starts around 85%, rises to 95% after 5 years, then drops.]
- [A simple table.]
5 years 81% 10 years 77%
- [Cueball and Megan are sitting on a bench, next to an IV drip hanging from a rack. Cueball is holding a paper.]
- Cueball: You know, probability used to be my favorite branch of math
- Cueball: Because it had so many real-life applications.
- [They embrace, faces together.]
Discussion
I've been through this, even though it was a little over a year ago, this strip brings me back to how I tried to rationalise the probabilities to deal with the news, and the only thing I could think of was "I want a better number, god, noodle-monster, anybody, please, give me a better number".
John 60.225.31.6 00:40, 28 September 2013 (UTC)
Can anyone please provide an update to this page on Randall's fiance's health? Apologies if this is common knowledge. I agree with John's reaction. I wanted a better number for my wife back in the spring of 2008, and got it. She survived 3 years instead of the (then) predicted average of six months for inflammatory breast cancer. We could have been just an outlier on the low probability end of the curve, but I like to think the medical community is continually improving their curves, and I am very grateful for the extra time. She passed away four days after this strip was posted - which explains why I haven't seen this strip until now.
108.162.216.228 21:26, 28 November 2013 (UTC)Grant
So, how is this incomplete? Can we remove the incompleteness mark? --173.245.53.196 13:02, 19 January 2014 (UTC)
I think the linking to the blog post is more sensitive than explaining it here. Remove tag? --141.101.98.207 14:19, 19 January 2014 (UTC)
What is the dashed line? It kind of looks like it might be the derivative of the solid line.108.162.214.53 00:39, 20 January 2014 (UTC)
I think I explained the dashed line, it's a hazard function or at least it would be a plausible hazard function for that kind of survival function. Feel free to improve the formatting or remove the incomplete tag. --Artod (talk) 07:06, 20 January 2014 (UTC)
TEN YEARS 172.69.33.179 17:38, 17 November 2020 (UTC)
